In this project we analyze the calculation of the. On computational aspects of Tchebichef polynomials for higher polynomial order IEEE Access 5 2017 24702478.
Discuss some computational aspects of Tchebichef polynomials and moments such as symmetry property polynomial expansion and recurrence relations with.
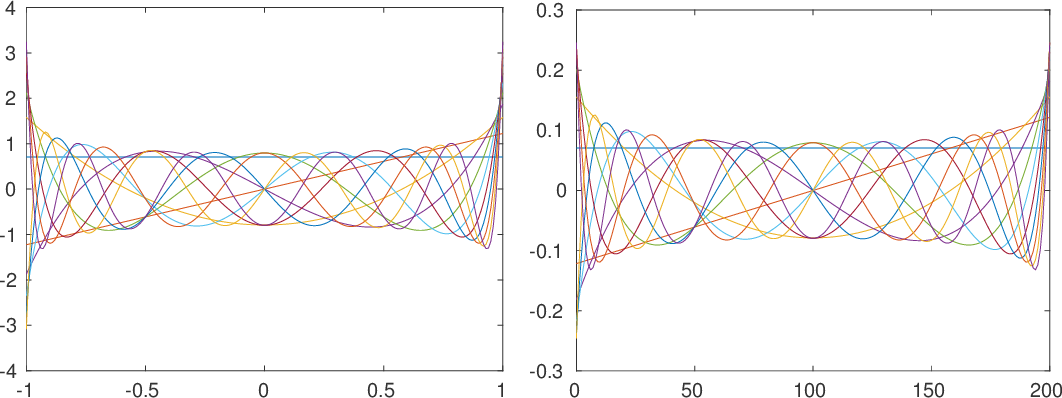
. Mukundan et al. The first type is orthogonal on a uniform lattice such as the Tchebichef Krawtchouk Hahn Meixner and. The proposed algorithm makes the computation of the TP superior to that of conventional recurrence algorithms when the polynomial order is large and also accelerates the.
Discrete orthogonal moments are utilized. PDF - Charlier polynomials CHPs and their moments are commonly used in image processing due to their salient performance in the analysis of signals and their capability in signal. On Computational Aspects of Tchebichef Polynomials for Higher Polynomial Order Published in IEEE Access in 2017 Web of Science Free Access.
Two types of discrete orthogonal polynomials can be distinguished. The major issue of CHPs is the numerical instability of coefficients for high-order polynomials. Rahman Ramli Syed Abdul Rahman Al-Haddad Basheera M.
One can find in the literature methods for numerically approximating the zeros of. In this study a new recurrence algorithm is proposed to generate CHPs for high-order. To overcome this problem Camacho-bello et al.
Abdulhussain Abd Rahman Ramli Syed Abdul Rahman Al-Haddad Basheera M. The calculation of the higher order polynomial base causes numerical instability seriously. On Computational Aspects of Tchebichef Polynomials for Higher Polynomial Order Sadiq H.
On Computational Aspects of Tchebichef Polynomials for Higher Polynomial Order. In Mukundan presented a seminal paper where the symmetry and renormalization of the Tchebichef polynomials are used to reduce the accumulation of numerical errors. In the past decades orthogonal moments OMs have received a significant attention and have widely been applied in various applications.
The high-order orthogonal polynomial base causes a numerical instability that makes it difficult to calculate the moments. Discrete Krawtchouk polynomials are widely utilized in different fields for their remarkable characteristics specifically the localization property. OMs are considered beneficial and.
On computational aspects of Tchebichef polynomials for higher polynomial order SH Abdulhussain AR Ramli SAR Al-Haddad BM Mahmmod WA Jassim IEEE Access 5 1. This is the second article on several computational aspects of polynomials in the ring. This article proposes a new method for the fast and efficient calculation of 3D Tchebichef momentswhich are an essential tool for the characterization and analysis of 3D objects.
25 Camacho-Bello C Rivera-Lopez JS Some computational. 5 propose the computation of higher-order Tchebichef polynomials via the modified Gram-Schmidt orthonormalization. Tchebichef moments use the Tchebichef polynomials as the kernel.
Abstract Charlier polynomials CHPs and their moments are commonly used in image processing due to their salient performance in the analysis of signals and their capability in. We observe that the Chebyshev polynomials form an orthogonal set on the interval 1 x 1 with the weighting function 1 x2 12 Orthogonal Series of Chebyshev Polynomials An arbitrary.

Discrete Chebyshev Polynomials Semantic Scholar
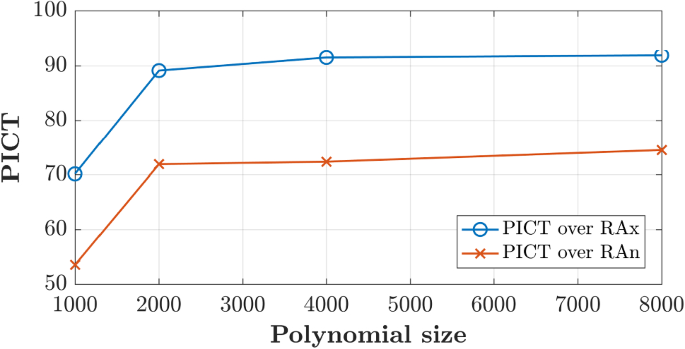
Fast And Efficient Recursive Algorithm Of Meixner Polynomials Springerlink

Discrete Chebyshev Polynomials Semantic Scholar

Plot Of Generalized Laguerre Polynomials For A 0 5 A 0 A 0 5 Download Scientific Diagram
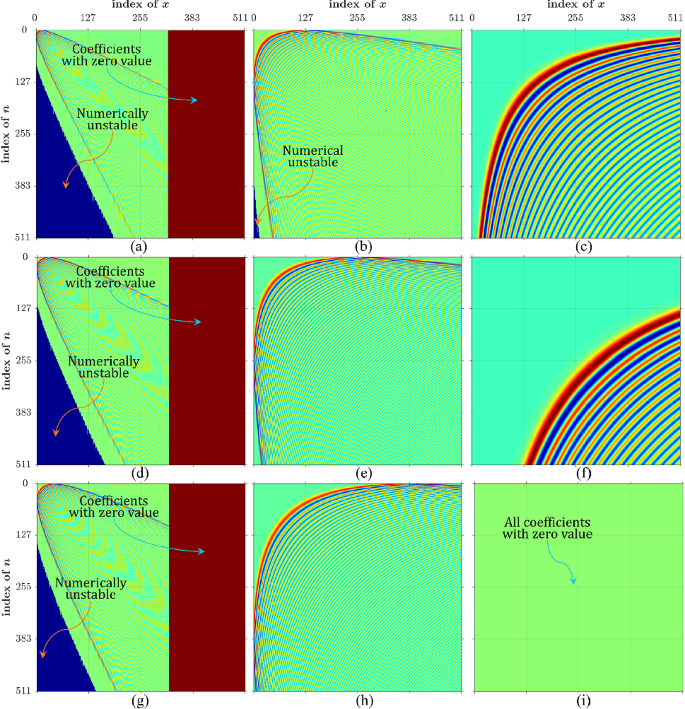
J Imaging Free Full Text On Computational Aspects Of Krawtchouk Polynomials For High Orders Html
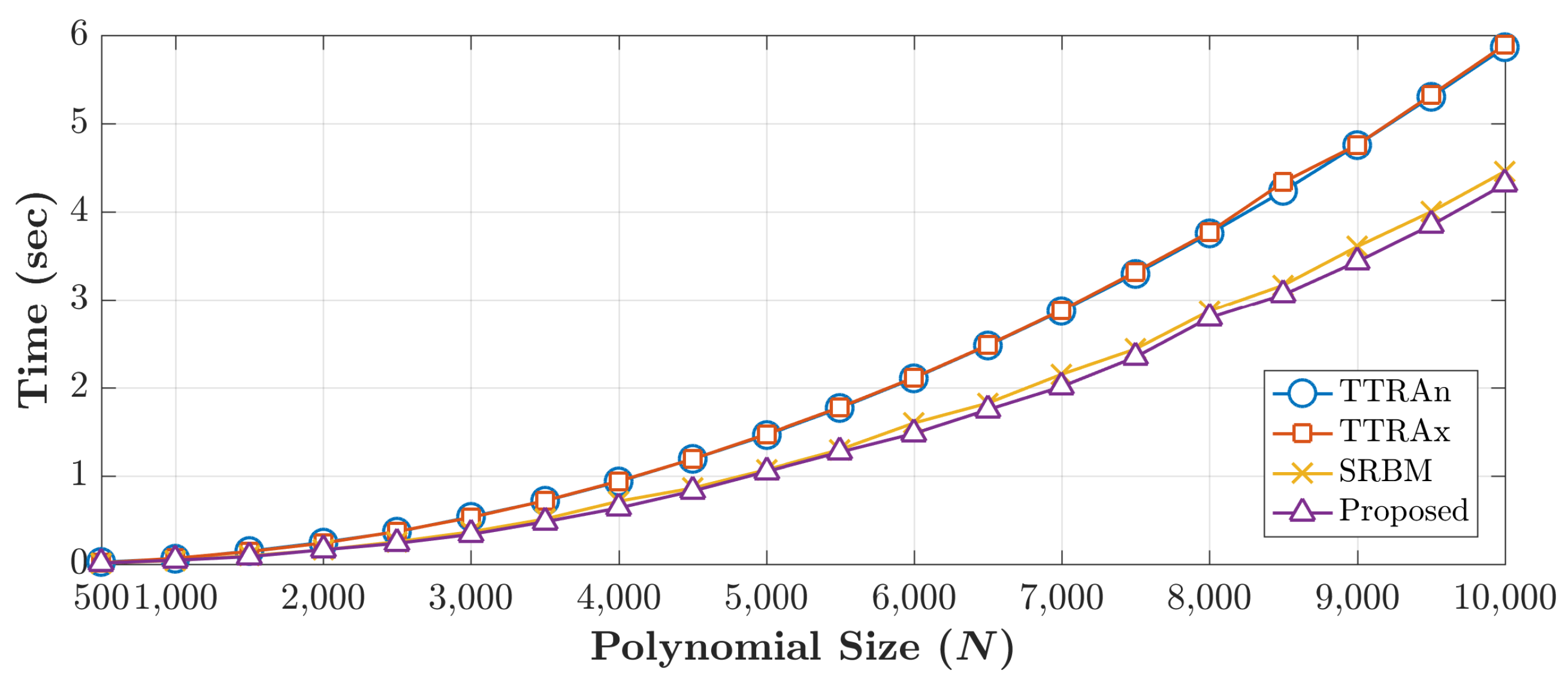
Fast And Efficient Recursive Algorithm Of Meixner Polynomials Springerlink

Discrete Chebyshev Polynomials Semantic Scholar

1d Signal To 2d Matrix Conversion Download Scientific Diagram
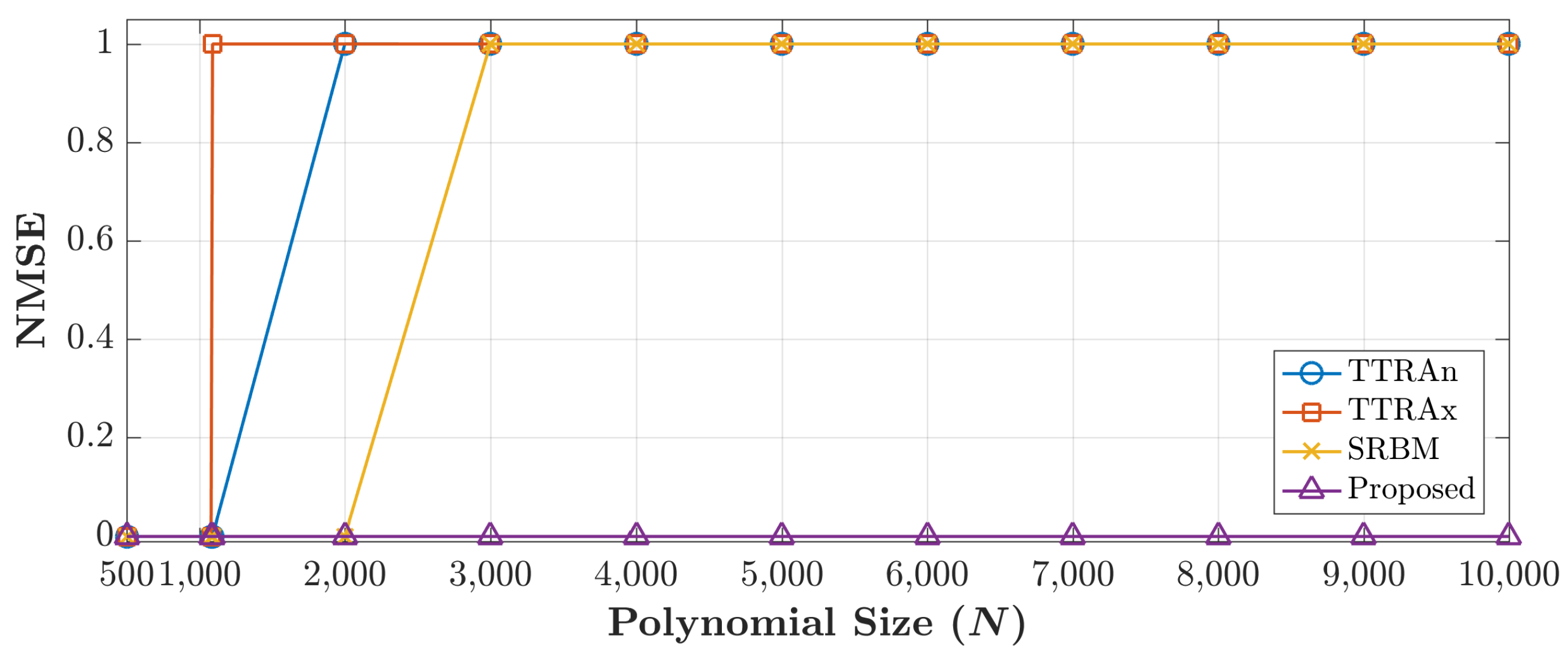
J Imaging Free Full Text On Computational Aspects Of Krawtchouk Polynomials For High Orders Html
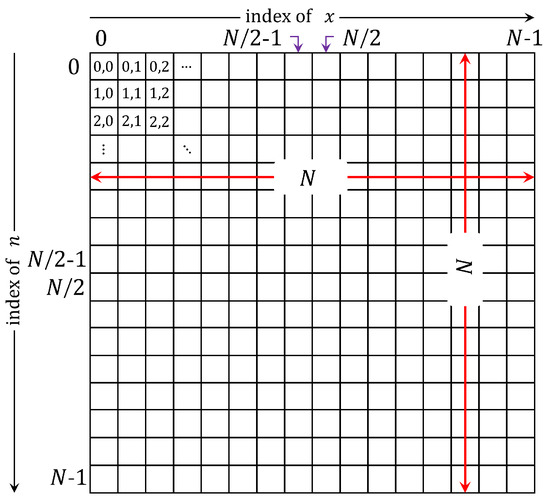
J Imaging Free Full Text On Computational Aspects Of Krawtchouk Polynomials For High Orders Html
